transmission line impedance calculator

Introduction to Transmission line impedance

Transmission lines are essential components in electrical power systems, facilitating the efficient transfer of electrical energy from power generation sources to consumers. Understanding the impedance characteristics of transmission lines is crucial for designing, analyzing, and optimizing power networks. This article delves into the concept of transmission line impedance and provides a comprehensive guide on using a transmission line impedance calculator.

What is Transmission Line Impedance?

Transmission line impedance refers to the total opposition a transmission line offers to the flow of alternating current (AC). It is a complex quantity that consists of both resistance and reactance. The impedance of a transmission line depends on various factors, including the line’s physical properties, such as conductor material, cross-sectional area, and spacing between conductors, as well as the operating frequency and environmental conditions.

Importance of Calculating Transmission Line Impedance

Accurate calculation of transmission line impedance is vital for several reasons:

1. Power Flow Analysis: Impedance values are used in power flow studies to determine the distribution of power and voltage levels throughout the power system.

2. System Stability: Transmission line impedance influences the stability of the power system, affecting factors such as voltage regulation, power transfer capability, and fault current levels.

3. Protection System Design: Impedance data is essential for designing and setting protective devices, such as relays and circuit breakers, to ensure reliable and safe operation of the power network.

4. Transmission Line Design: Impedance calculations are necessary for selecting appropriate conductor sizes, determining tower configurations, and optimizing the overall design of transmission lines.

Transmission Line Impedance Components

Transmission line impedance consists of two main components: resistance and reactance.

Resistance (R)

Resistance is the opposition to the flow of electric current due to the inherent properties of the conductor material. It is primarily determined by the conductor’s material, cross-sectional area, and length. The resistance of a transmission line is affected by factors such as temperature and skin effect.

Factors Affecting Resistance

1. Conductor Material: The resistivity of the conductor material directly impacts the resistance. Common conductor materials used in transmission lines include aluminum, copper, and aluminum-conductor steel-reinforced (ACSR).

2. Cross-Sectional Area: A larger cross-sectional area of the conductor results in lower resistance, as it provides more pathways for current flow.

3. Length: The resistance of a transmission line is directly proportional to its length. Longer lines have higher resistance compared to shorter lines.

4. Temperature: The resistance of a conductor increases with temperature. As the temperature rises, the increased thermal agitation of electrons impedes current flow, leading to higher resistance.

5. Skin Effect: At high frequencies, the current tends to flow more densely near the surface of the conductor, effectively reducing the cross-sectional area and increasing the resistance. This phenomenon is known as the skin effect.

Reactance (X)

Reactance is the opposition to the flow of alternating current due to the inductive and capacitive properties of the transmission line. It consists of two components: inductive reactance (XL) and capacitive reactance (XC).

Inductive Reactance (XL)

Inductive reactance arises from the magnetic field produced by the current flowing through the transmission line. It is proportional to the frequency of the alternating current and the inductance of the line. Inductance is determined by factors such as the spacing between conductors, the presence of ground wires, and the permeability of the surrounding medium.

Capacitive Reactance (XC)

Capacitive reactance occurs due to the electric field between the conductors of the transmission line. It is inversely proportional to the frequency of the alternating current and the capacitance of the line. Capacitance is influenced by factors such as the spacing between conductors, the diameter of the conductors, and the permittivity of the insulating medium.

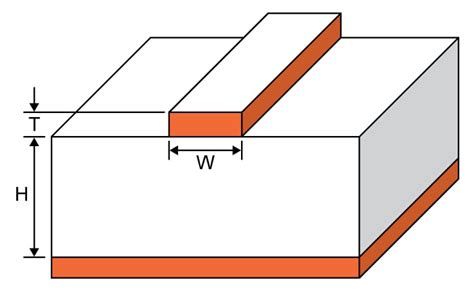
Transmission Line Impedance Calculation

To calculate the impedance of a transmission line, several parameters need to be considered. The following sections discuss the steps involved in impedance calculation.

Step 1: Gather Transmission Line Data

To calculate the impedance of a transmission line, you need to gather the following data:

1. Conductor Material: Determine the material of the conductors used in the transmission line (e.g., aluminum, copper, ACSR).

2. Conductor Size and Configuration: Obtain information about the conductor size (cross-sectional area) and the configuration of the conductors (e.g., single circuit, double circuit, bundle conductors).

3. Line Length: Measure the total length of the transmission line.

4. Conductor Spacing: Determine the spacing between the conductors and the spacing between phases (for multi-phase lines).

5. Ground Wire Details: If ground wires are present, obtain information about their size, material, and configuration.

6. Operating Frequency: Identify the frequency at which the transmission line operates (e.g., 50 Hz, 60 Hz).

Step 2: Calculate Resistance (R)

To calculate the resistance of the transmission line, use the following formula:

R = ρ × (L / A)

Where:
– R is the resistance (Ω)
– ρ is the resistivity of the conductor material (Ω·m)
– L is the length of the transmission line (m)
– A is the cross-sectional area of the conductor (m²)

The resistivity (ρ) of common conductor materials at 20°C is given below:

Material	Resistivity (Ω·m)
Aluminum	2.82 × 10⁻⁸
Copper	1.68 × 10⁻⁸
ACSR	3.18 × 10⁻⁸

Step 3: Calculate Inductive Reactance (XL)

The inductive reactance of a transmission line can be calculated using the following formula:

XL = 2π × f × L

Where:
– XL is the inductive reactance (Ω)
– f is the operating frequency (Hz)
– L is the inductance of the transmission line (H)

The inductance (L) of a transmission line depends on the conductor configuration and spacing. For a single-phase line with two parallel conductors, the inductance can be approximated using the following formula:

L = (μ₀ / 2π) × ln(D / r)

Where:
– L is the inductance per unit length (H/m)
– μ₀ is the permeability of free space (4π × 10⁻⁷ H/m)
– D is the spacing between conductors (m)
– r is the radius of the conductor (m)

For more complex conductor configurations, such as multi-phase lines or bundle conductors, specialized formulas or software tools are used to calculate the inductance accurately.

Step 4: Calculate Capacitive Reactance (XC)

The capacitive reactance of a transmission line can be calculated using the following formula:

XC = 1 / (2π × f × C)

Where:
– XC is the capacitive reactance (Ω)
– f is the operating frequency (Hz)
– C is the capacitance of the transmission line (F)

The capacitance (C) of a transmission line depends on the conductor configuration, spacing, and the permittivity of the insulating medium. For a single-phase line with two parallel conductors, the capacitance can be approximated using the following formula:

C = (2π × ε₀) / ln(D / r)

Where:
– C is the capacitance per unit length (F/m)
– ε₀ is the permittivity of free space (8.85 × 10⁻¹² F/m)
– D is the spacing between conductors (m)
– r is the radius of the conductor (m)

Similar to inductance calculations, specialized formulas or software tools are used for more complex conductor configurations.

Step 5: Calculate Total Impedance (Z)

The total impedance of the transmission line is the sum of the resistance and the reactive components. It is represented as a complex number:

Z = R + j(XL – XC)

Where:
– Z is the total impedance (Ω)
– R is the resistance (Ω)
– XL is the inductive reactance (Ω)
– XC is the capacitive reactance (Ω)
– j is the imaginary unit (√-1)

The magnitude of the impedance (|Z|) can be calculated using the following formula:

|Z| = √(R² + (XL – XC)²)

The phase angle (θ) of the impedance can be calculated using:

θ = arctan((XL – XC) / R)

Using a Transmission Line Impedance Calculator

To simplify the process of calculating transmission line impedance, various online tools and software programs are available. These calculators provide a user-friendly interface for inputting transmission line parameters and generate accurate impedance results.

Steps to Use a Transmission Line Impedance Calculator

1. Enter the necessary transmission line data, such as conductor material, size, configuration, line length, and operating frequency.

2. Specify the spacing between conductors and any additional details like the presence of ground wires.

3. Select the desired units for input and output values (e.g., kilometers, miles, ohms).

4. Click the “Calculate” or “Submit” button to obtain the impedance results.

The calculator will display the resistance (R), inductive reactance (XL), capacitive reactance (XC), and total impedance (Z) of the transmission line. Some calculators may also provide additional information, such as the impedance per unit length or the impedance at different temperatures.

Benefits of Using a Transmission Line Impedance Calculator

1. Time-saving: Impedance calculators eliminate the need for manual calculations, saving time and effort.

2. Accuracy: Calculators use precise formulas and consider various factors, ensuring accurate impedance results.

3. User-friendly: Most calculators have intuitive interfaces, making them accessible to users with varying levels of technical expertise.

4. Flexibility: Calculators allow users to quickly modify input parameters and observe the impact on impedance values, facilitating sensitivity analysis and optimization.

Conclusion

Transmission line impedance is a critical parameter in power system analysis, design, and operation. It consists of resistance and reactance components, which are influenced by factors such as conductor material, size, spacing, and operating frequency. Calculating transmission line impedance involves gathering necessary data and applying appropriate formulas for resistance, inductive reactance, and capacitive reactance.

To streamline the impedance calculation process, transmission line impedance calculators are widely used. These tools provide a convenient and accurate means of determining impedance values based on user inputs. By understanding the concepts behind transmission line impedance and utilizing impedance calculators, power system engineers and designers can make informed decisions, optimize power flow, and ensure the reliable operation of electrical networks.

Frequently Asked Questions (FAQ)

1. What is the purpose of calculating transmission line impedance?
   Answer: Calculating transmission line impedance is essential for power flow analysis, system stability studies, protection system design, and transmission line design. It helps determine the distribution of power, voltage levels, fault currents, and overall performance of the power system.

2. What are the main components of transmission line impedance?
   Answer: Transmission line impedance consists of two main components: resistance (R) and reactance (X). Reactance further comprises inductive reactance (XL) and capacitive reactance (XC). The total impedance (Z) is the sum of resistance and the reactive components.

3. How does the conductor material affect the resistance of a transmission line?
   Answer: The conductor material’s resistivity directly impacts the resistance of a transmission line. Materials with lower resistivity, such as copper, have lower resistance compared to materials like aluminum or ACSR (aluminum-conductor steel-reinforced). The choice of conductor material is based on factors such as cost, weight, and conductivity.

4. What is the skin effect, and how does it influence transmission line impedance?
   Answer: The skin effect is a phenomenon that occurs at high frequencies, where the current tends to flow more densely near the surface of the conductor. This effectively reduces the cross-sectional area available for current flow and increases the resistance of the transmission line. The skin effect becomes more pronounced as the frequency increases.

5. Can transmission line impedance calculators handle complex conductor configurations?
   Answer: Yes, many transmission line impedance calculators are designed to handle complex conductor configurations, such as multi-phase lines or bundle conductors. These calculators often incorporate specialized formulas or numerical methods to accurately determine the impedance values for various configurations. However, it is important to ensure that the calculator being used is suitable for the specific transmission line configuration under consideration.